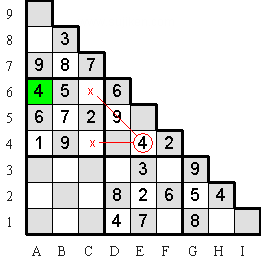
A 4 must appear in the middle-left square and it must be placed in A6.
Why? Because the 4 in E4 prevents a 4 from being placed in C4 or
C6.
Principle: Pay attention to the diagonals when placing digits.
A 9 must appear in the lower square and you can place it in F1
because of the 9 in G3.
Principle: A 3x3 Square region must contain all nine digits.
The lower square must contain a 5 and it must be
placed in D3 because the 5 in G2 prevents a 5 from being placed in
F3.
Principle: Pay attention to the diagonals when placing digits.
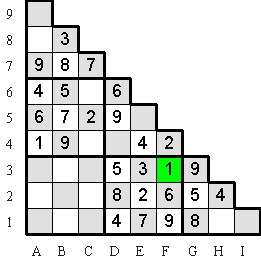Complete the lower square by placing its missing digit, 1, in F3.
Principle: A 3x3 Square region must contain all nine digits.
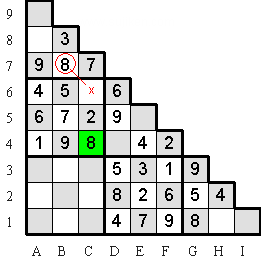The middle-left square needs an 8 and it must be placed in C4 because of the 8
in B7.
Principle: Pay attention to the diagonals when placing digits.
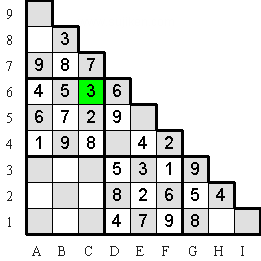Complete the middle-left square by placing its missing digit,
3, in C6.
Principle: A 3x3 Square region must contain all nine digits.
The lower-left square needs an 8 and it must be placed in A3 because of
C4, D2
and G1.
Principle: When a digit has been eliminated from all other eight cells within a region with nine cells, then you have determined the correct digit.
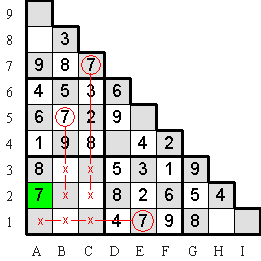The lower-left square needs a 7 and it must be placed in A2 because of
B5, C7 and E1.
Principle: When a digit has been eliminated from all other eight cells within a region with nine cells, then you have determined the correct digit.
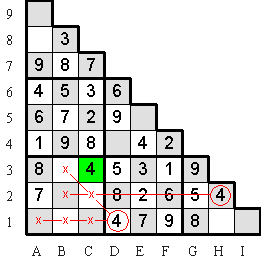The lower-left square needs a 4 placed in C3 because of
D1 and
H2.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
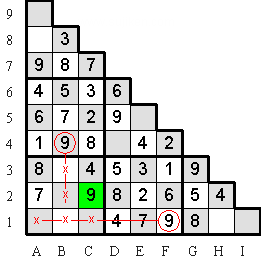The lower-left square needs a 9 and it must be placed in C2 because of B4 and
F1.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
The lower-left square needs a 3 and it must be placed in A1 because of
B8
and C6.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
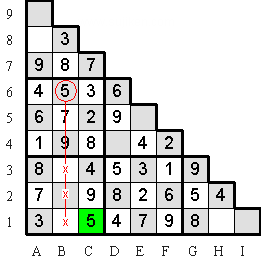The lower-left square
needs a 5 and it must be placed in
C1 because of
B6.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
Column A must contain
all nine digits. Its two missing digits are {2, 5}. Because of the 2 in F4,
A9 cannot contain a 2, therefore you must place a 2 in A8.
Principle: Take advantage of the the fact that Column A must contain nine unique digits.
Complete column A by placing the last digit, 5, in A9.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
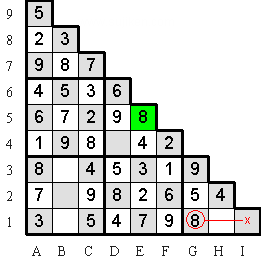The long diagonal
from A9 to I1 must contain 9 digits, thus its two missing digits are {1, 8}.
Because of the 8 in G1, I1 cannot contain an 8, therefore you must
place an 8 in E5.
Principle: Take advantage of the fact that the long diagonal from A9 to I1 must contain nine unique digits.
Complete this long diagonal
by placing the last digit, 1, in
I1.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
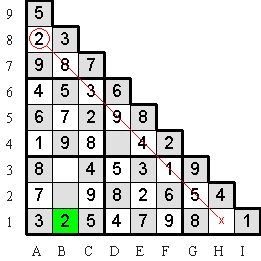Row 1
must contain 9 digits, so its two missing digits are {2, 6}. Because of the 2 in A8,
H1 cannot contain a 2, therefore you must place a 2 in
B1.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
Complete row 1 by
placing the last digit, 6, in H1..
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
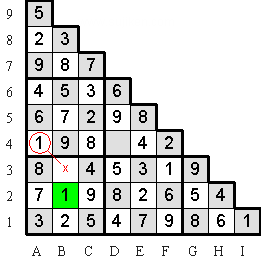The lower-left square must contain a 1 in B2 because of A4.
Principle: Pay attention to the diagonals when placing digits.
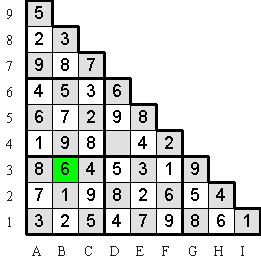Complete the lower-left square by placing its last digit, 6, in B3.
Principle: Take advantage of the regions that must contain nine digits: The upper-left square, lower-left square, lower square, Row 1, Column A and the long diagonal from A9 to I1.
If you consider the diagonal from A7 to G1, you
will see that D4 cannot contain a {2, 3, 5, 6, 8, 9}; D4 cannot contain
a {1, 4} because of the diagonal from A1 to E5. Thus D4
must contain a 7.
Principle: If you eliminate eight digits from being placed in a cell, then the remaining ninth digit must be placed.
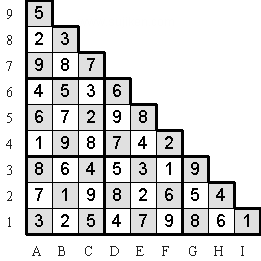No digit
is duplicated in any horizontal row, vertical column or diagonal. This property
holds regardless of the number of cells in the row, column or diagonal. The diagonal from A9 to I1 has nine unique digits
while a shorter
diagonal from B1 to E4 has four
unique digits. Note, too,
that the square and triangular regions contain no duplicate digit.We hope this tutorial was helpful to you! Now good luck with your SujikenTM puzzles!